Wind Load Example
(How to calculate a Wind Load)
by www.reasonablepower.com

Generally we start with a question, such as:
What is the wind load on a flat plate, which is stationary in the wind, which is round, which has a diameter of 14 ft (or 7 ft radius), and which has a 50 mile per hour wind hitting it?
ANSWER:

Area = A = (pi)(radius)(radius) = (3.1415926535) x (7 ft) x (7 ft)
Area = A = 153.9380400215 square feet
or in SI units (1 square foot = 0.09290304 square meter); Area = 14.3013118896 square meter
Wind Speed = v = 50 mph
or in SI units (1 mile/hour = 0.44704 meter/second); Wind Speed = 22.352 meter/second
Using the formula:

*(Note the other formulas after this article.)
Where
F = Force = Fd = is the
force against ANY surface, and it is the
force against ANY shape, or the drag created by the force against ANY surface or shape (or in this example the Force Against the flat plate), but it is applicable to ANY surface and applicable to ANY shape whether as "force against that shape or surface" or as "drag caused by that shape or surface".
This formula should be used to accurately calculate the "force against" or the "drag caused by" wind powered generators, or those silly illogical waste-of-space wind turbines, or airplanes, or kites (even complicated kites with multiple morphing shapes) and the strings holding those kites, or hang-gliders (powered or un-powered), or bullets, or birds, or parts falling off of satellites passing through the air, or any object whatsoever. No exceptions. This formula works for ALL shapes moving or tumbling or spinning or solidly held.
We are using "Fd" as the force instead of "F" because it is in a commonly found formula. F is the same as Fd and both are equal to the
force of drag ("Fd") (or in this case Force Against ("F") the flat plate). Same thing in this case.
p is the
density of the air,
v is the
speed of the air against the object,
A is the
area of the object which the air is blowing against,
Cd is the
drag coefficient,
The
density of air at sea level is about 1.2 kg/m^3 (1.2 kilo grams per cubic meter) .
p = 1.2 kilogram/cubic meter (for this example)
The
drag coefficient for a flat plate in open air is about 1.28
Cd (which is a dimensionless constant)= 1.28 (for this example)
Thus:
Fd = (1/2)(1.2 kilograms/cubic meter)(22.352 meter/second)(22.352 meter/second)(14.3013118896 square meter)(1.28)
-
Fd = Wind load on the 14 foot diameter flat plate = 5,487.44114 kilogram meter / second^2 = 5,487.44114 Newtons
-
We can show this as a "poundal" (or "pdl") which is a unit of acceleration defined as the force necessary to accelerate 1 pound mass an additional velocity of 1 foot per second, each second.
1 Newton = 7.233013851 poundal.
Converting: Fd = (5,487.44114 Newtons)(7.233013851 poundal / Newton) = 39,690.7377721672 poundal. (Rounding to 39,690.73)
-
We can show this as a "pound-force" (or "lbf") which is a unit of acceleration defined as the force necessary to accelerate 1 pound of mass an additional velocity of 32.17405 feet per second, each second.
1 Newton = 0.224808943 pound-force.
Converting: Fd = (5,487.44114 Newtons)(0.224808943 pound-force / Newton) = 1,233.62584245811502 pound-force. (Rounding to 1,233.62)
Notice that a poundal (pdl) is VERY different from a pound-force (lbf).
A poundal uses a velocity change of 1 foot per second.
A pound-force uses a velocity change of 32.17405 feet per second.
VERY BIG DIFFERENCE !
When you read through this example, remember that difference.
If (for example) we are working with a wind powered generator which has 4' long blades, and each blade starts at 3' from the center, we want to subtract the Fd of a 6' diameter flat plate from the Fd of the 14' diameter flat plate.
First we substitute the areas:
Area = A = (pi)(3 ft)(3 ft) = 28.274333882 square feet
or in SI units (1 square foot = 0.09290304 square meter); Area = 2.626771572 square meters
Fd = (1/2)(1.2 kilograms/cubic meter)(22.352 meter/second)(22.352 meter/second)(2.6267 square meter)(1.28)
Fd = 1,007.8973 kilogram meter / second^2 , which is the same as 1,007.8973 Newtons
or if we go back to previous units (1 Newton = 7.233013851 pound foot/square second);
Fd = 7,290.1355 pound foot / second^2
-
Fd = Wind load on the 6 foot diameter flat plate = 1,007.8973 kilogram meter / second^2 = 1,007.8973 Newtons
-
In poundal:
1 Newton = 7.233013851 poundal.
Converting: Fd = (1,007.8973 Newtons)(7.233013851 poundal / Newton) = 7,290.1351312855023 poundal. (Rounding to 7,290.13)
-
In pound-force:
1 Newton = 0.224808943 pound-force.
Converting: Fd = (1,007.8973 Newtons)(0.224808943 pound-force / Newton) = 226.5843266655539 pound-force. (Rounding to 226.58)
Then we subtract the two forces: (The wind load on the 14 foot diameter flat plate) - (The wind load on the 6 foot diameter flat plate)
The wind load on the 14 foot diameter flat plate was calculated as 5,487.44114 Newtons.
The wind load on the 6 foot diameter flat plate was calculated as 1,007.8973 Newtons.
Subtracting: (5,487.44114 Newtons) - (1,007.8973 Newtons) = 4,479.54384 Newtons
-
Fd = Wind load on the 14 foot diameter flat plate which has a 6 foot diameter hole in the middle = 4,479.54384 Newtons.
-
In poundal:
1 Newton = 7.233013851 poundal.
Converting: Fd = (4,479.54384 Newtons)(7.233013851 poundal / Newton) = 32,400.60264088172784 poundal. (Rounding to 32,400.60)
-
In pound-force:
1 Newton = 0.224808943 pound-force.
Converting: Fd = (4,479.54384 Newtons)(0.224808943 pound-force / Newton) = 1,007.04151579256112 pound-force. (Rounding to 1,007.04)
This 32,400 poundals is the force on the blades if the blades are being held still. When the blades turn, at different speeds there is different force on the blades. For now, we are simply looking at the force on the blades while they are stationary.
We can use this in both calculating the wind load and in calculating the center shaft torque for horsepower.
If (for example) we are working with a wind powered generator which has it's blades (currently) set at a 45 degree angle, then (in an ideal theoretical situation) we could use the hypotenuse formula and a little bit of trigonometry for right triangles to calculate the turning force (this is actually not "lift" but rather it is the force in the plane of the blade sweep circle and tangent to the blade sweep radius) and the drag force. The turning force would be (the wind load at 90 degrees, which is the hypotenuse) multiplied by (the sine of 45 degrees) = (4,479.54384 Newtons)x(0.70711) = 3,167.5302447024 Newtons. Since the blades are set at a 45 degree angle, the turning force is the same amount as the drag force. Notice that the wind load against the blades, which are not yet being allowed to turn, is now 3,167.5302447024 Newtons instead of 4,479.54384 Newtons because the blades are on an angle.
(3,167.5302447024 Newtons)(3,167.5302447024 Newtons) + (3,167.5302447024 Newtons)(3,167.5302447024 Newtons) = (10,033,247.85) + (10,033,247.85) = 20,066,495.70 Newtons.
The square root of 20,066,495.70 = 4,479.5642310385504729018488607803 , or rounded it is 4,479.56 (Newtons) which is very close to (remember we are rounding) the wind load at 90 degrees, so it is correct.
We rounded our numbers and estimated as we went along in this example, so close is fine.
Thus:
We have about 4,479.56 Newtons (spread out over different distances from the center) attempting to turn the blades. That is a lot!
And because the angle of the blades is set at 45 degrees, we also have about 4,479.56 Newtons of wind load.
Cantilever Torque
Units of measure:
Newton Meters
, or
poundal foot
, or
foot pound-force
.
If (for example) we are working with a wind powered generator which has it's blades centered at 100' above the ground, to get the cantilever torque (of the blades, and only the blades) which is attempting to bend the support at it's base, we multiply the wind load against the height.
That, in this example, is the wind load of the blades (set at a 45 degree angle), and it does not include the support, nor any of the structure.
Cantilever Torque (by the blades alone while they are still stationary and while they are being hit by a 50 mile per hour wind) attempting to bend the support at it's base = Tc = Force x Distance
Tc = Force x Distance
Lets look at this first in Newtons.
The Force is the wind load of 4,479.56 Newtons.
The Distance is 100 feet (to the center of the blades). Converting: (100 feet)(0.3048 meter / foot) = 30.48 meters.
Tc = (4,479.56 Newtons)(30.48 meters) = 136,536.9888 Newton Meters.
-
Tc = 136,536.98 Newton Meters
-
In poundal foot:
1 Newton Meter = 23.730366088 poundal foot.
Converting: Tc = (136,536.9888 Newton Meters)(23.730366088 poundal foot / Newton Meter) = 3,240,072.7287771558144 poundal foot. (Rounding to 3,240,072.72)
-
In foot pound-force:
1 Newton Meter = 0.737562121 foot pound-force
Converting: Tc = (136,536.98 Newton Meter)(0.737562121 foot pound-force / Newton Meter) = 100,704.50456373458 foot pound-force. (Rounding to 100,704.50)
In the USA we commonly use foot pound-force in describing torque.
Thus we would say that
-
The wind load is 100,704.50 "foot pound-force".
And as has been pointed out, saying 100,704.50 "pound-force" (instead of "foot pound-force") is incorrect because of the units.
-
The torque attempting to bend the support pole at its base is 100,704.50 "foot pound-force".
We therefore can see that there is a lot of energy involved in the use of even a small Wind Powered Generator such as in this example.
If (for example) we want to know how much torque the wind load against the blades generates against the shaft, while the blades are not yet being allowed to turn, we spread out the force per area of the blades and per distance from the center of the shaft and calculate that.
A little example of this,
calculated at a lower wind speed (from 1 mph to 5 mph) is:
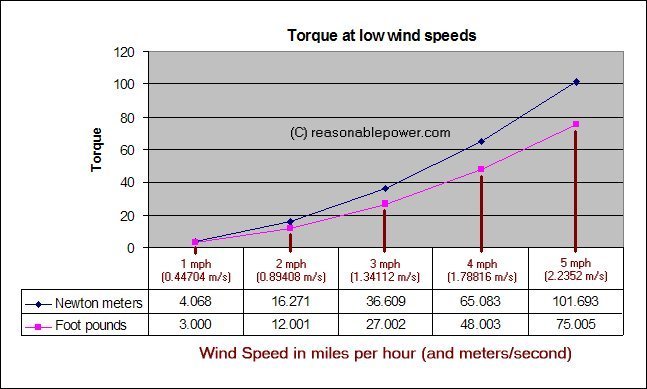
As you can see, the torque on the shaft,
. . at
. .
LOW WIND SPEED
. . . . is
. .
quite powerful already.
Remember that at this point we have not included occasional wind shear, nor resistance of the bearings, etc.







 *(Note the other formulas after this article.)
*(Note the other formulas after this article.)

